What Are Some Examples in Which the Pythagorean Theorem Is Used in Real Life?
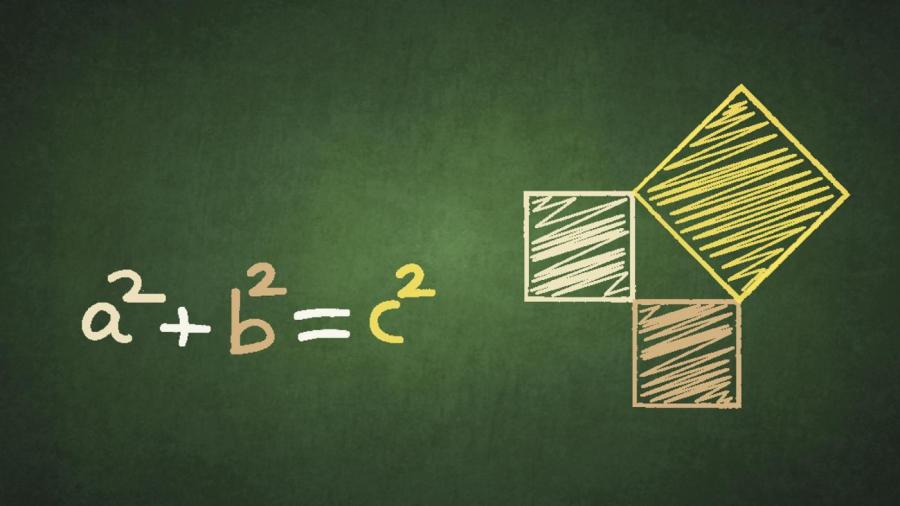
The Pythagorean theorem is used often in construction, in engineering, in architecture, in design, in art and in aeronautics. The theorem states that, given a right triangle with sides a, b and c, where c represents the hypotenuse (or longest side), the sum of the square of the two sides (a squared plus b squared) is always equal to c squared.
Most of the common use applications of the Pythagorean theorem relate to finding the shortest distance between two points. The hypotenuse of a right triangle is the diagonal line that bisects any regular rectangle, and the theorem is the only way of calculating the length of that diagonal without measuring. If someone is traveling 3 miles east, then 4 miles north, the length of a direct line from his starting point to his ending point is the square root of 3² plus 4², or 5 miles. If he is painting a wall 12 feet high, and the foot of the ladder needs to be 5 feet from the wall, then he knows he needs a 13-foot ladder, because the square root of 5² plus 12² equals 13². Physicists and engineers use the Pythagorean theorem all the time because right triangles are everywhere. Any time distance is plotted on a map, it involves right-angle relationships. Likewise, when calculating the height of an object, whether a wall or an airplane, the Pythagorean theorem makes it easy.





